Is 0 An Even Number? Understanding The Concept Of Even And Odd Numbers
The question of whether 0 is an even number has intrigued mathematicians and laypeople alike for centuries. In mathematics, numbers are categorized into two main groups: even numbers and odd numbers. Understanding these categories is crucial for mathematical operations and number theory. In this article, we will delve into the definition of even and odd numbers, explore the role of 0 in these classifications, and provide a comprehensive analysis of why 0 is indeed considered an even number.
To begin with, let's clarify the definitions of even and odd numbers. An even number is any integer that can be divided by 2 without leaving a remainder. Conversely, an odd number is an integer that, when divided by 2, has a remainder of 1. This fundamental understanding sets the stage for our exploration of the number 0.
In the following sections, we will examine the mathematical properties of 0, analyze its behavior in arithmetic operations, and present arguments from various mathematical perspectives. By the end of this article, readers will have a clear understanding of why 0 is classified as an even number, along with its significance in the broader context of mathematics.
Table of Contents
- Definition of Even and Odd Numbers
- Properties of Zero
- Mathematical Justifications for Zero as an Even Number
- Zero's Role in Arithmetic Operations
- Historical Context of Zero in Mathematics
- Educational Implications of Understanding Zero
- Common Misconceptions About Zero
- Conclusion
Definition of Even and Odd Numbers
Even and odd numbers are fundamental concepts in mathematics. Let's break down the definitions:
- Even Numbers: Any integer that is divisible by 2. Examples include -4, -2, 0, 2, 4, etc.
- Odd Numbers: Any integer that is not divisible by 2. Examples include -3, -1, 1, 3, etc.
From these definitions, we can see that an even number will yield a result of zero when divided by 2, while an odd number will not.
Properties of Zero
Zero (0) is a unique number with several distinct properties:
- Identity Element: In addition, 0 is the identity element because adding 0 to any number does not change its value.
- Divisibility: Zero is divisible by any non-zero integer, which supports its classification as an even number.
- Neutral Element: In multiplication, any number multiplied by 0 results in 0.
These properties play a crucial role in determining whether 0 fits into the category of even or odd numbers.
Mathematical Justifications for Zero as an Even Number
There are several mathematical justifications for classifying 0 as an even number:
- Definition Compliance: According to the definition of even numbers, since 0 divided by 2 equals 0 (with no remainder), it meets the criteria for being even.
- Number Line Position: On the number line, 0 is positioned between -1 and 1, directly aligning with other even numbers.
- Pattern Recognition: The sequence of even numbers (…), -4, -2, 0, 2, 4, … includes 0, reinforcing its classification.
Zero's Role in Arithmetic Operations
Zero plays a significant role in arithmetic operations:
- Addition: Adding 0 to any number yields the original number.
- Subtraction: Subtracting 0 from any number also yields the original number.
- Multiplication: Any number multiplied by 0 results in 0, emphasizing its unique characteristics.
- Division: Dividing by 0 is undefined, but 0 divided by any non-zero number equals 0.
These operations illustrate how 0 functions within the realm of even and odd numbers.
Historical Context of Zero in Mathematics
The concept of zero has a rich history in mathematics:
- Origin: Zero was first introduced by ancient Indian mathematicians and later adopted by Arab scholars.
- Symbolism: Initially, zero represented a placeholder in positional number systems, but its significance evolved over time.
- Acceptance: By the Renaissance, zero was widely accepted in mathematical literature, solidifying its place in number theory.
This historical context highlights the importance of understanding zero's classification as an even number.
Educational Implications of Understanding Zero
Understanding the classification of zero is essential in educational contexts:
- Mathematical Foundations: It lays the groundwork for more complex mathematical concepts.
- Critical Thinking: Encourages students to think critically about numerical classifications.
- Application: Helps students apply these concepts in real-world scenarios, such as computer science and engineering.
Common Misconceptions About Zero
Despite its classification as an even number, several misconceptions exist about zero:
- Zero is Neither Even Nor Odd: Some argue that since 0 is neutral, it cannot be classified. However, as discussed, it meets the criteria for even numbers.
- Zero is a Negative Number: Zero is neither positive nor negative, making it a distinct entity in number theory.
- Zero is Not a Real Number: Zero is indeed a real number and is crucial in various mathematical applications.
Conclusion
In summary, 0 is classified as an even number based on its compliance with the mathematical definition of evenness, its unique properties, and its significance in arithmetic operations. Understanding this classification enhances our comprehension of number theory and mathematics as a whole.
We encourage readers to engage with this topic further by leaving comments, sharing this article, or exploring related content on our site. Thank you for joining us in this mathematical exploration!
We hope you found this article enlightening and informative. Feel free to return for more intriguing discussions on mathematics and its many fascinating aspects!
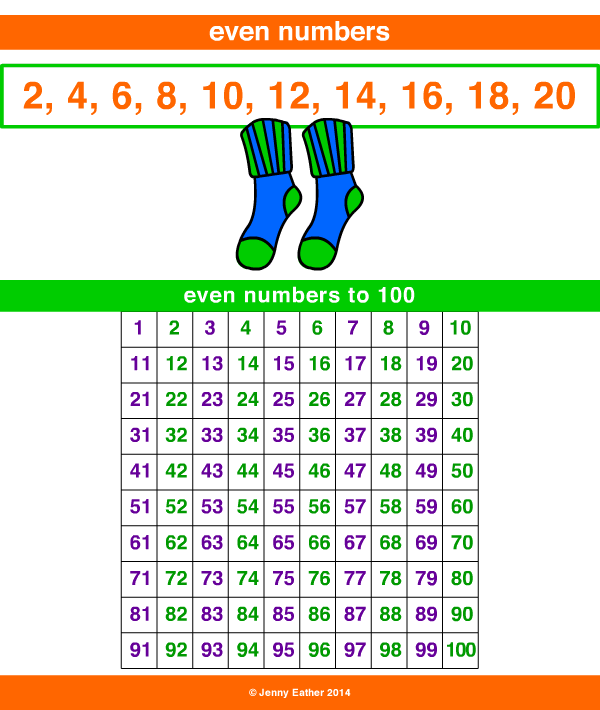
even A Maths Dictionary for Kids Quick Reference by Jenny Eather
regular expression for even number of 0's followed by odd number of 1's
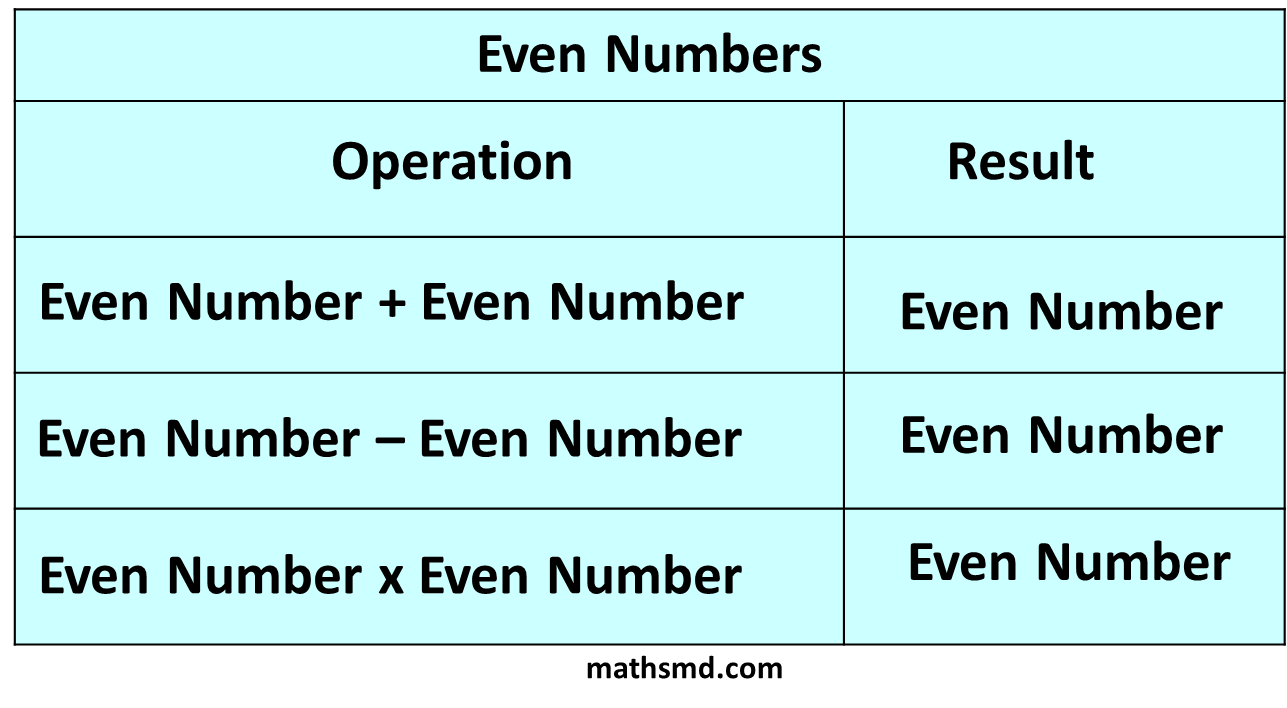
Even Numbers Properties and List of Even Numbers MathsMD